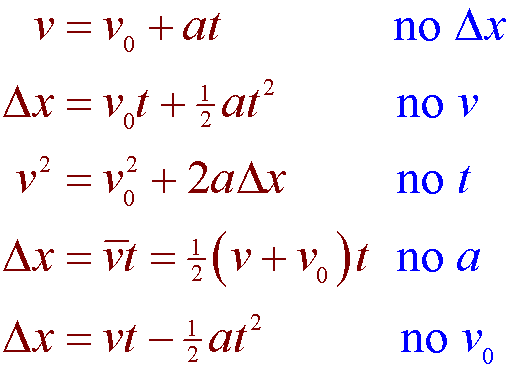Outrageous 3 Equations Of Motion

Derivation of Second Equation of Motion.

3 equations of motion. V2 v02 2 a s v02 v2 2 a s v02 19 ms2 2 80 ms2 66 m. We are a social enterprise working on a mission to make school learning interesting relevant and affordable to every child on this planet. Depending on the problem being examined the kinematics equations may need to be examined either before or after the kinetics equations.

Let us try to derive these equations by graphical method. Putting the equation 3 in equation 2 the following equation is obtained. Look out for the vid.

Hence we get the first equation of motion. Google Classroom Facebook Twitter. Write three equations of uniformly accelerated motion relating the initial velocity u final velocity v time t acceleration a and displacement S.

Equations of motion in physics are defined as equations that describe the behaviour of a physical system in terms of its motion as a function of time. Hamiltons equations of motion summarized in equations ref825-ref827 use either a minimal set of generalized coordinates or the Lagrange multiplier terms to account for holonomic constraints or generalized forces Q_jEXC to account for non-holonomic or other forces. Three Equations of Motion The equations that relate displacement S time taken t initial velocity u final velocity v and uniform acceleration a are called equations of motion.

The three equations of motion are known as kinematic equations. These equations govern the motion of an object in 1D 2D and 3D. An object is in motion with initial velocity u attains a final velocity v in time t due to acceleration a with displacement s.

I derive all 4 equations of motion then go over some important points to remember when using them. V 2 v 0 2 2as s 0 3. It is already known that acceleration a is the rate of change of the velocity v.